The Bimodal Magnetosphere and Radiation Belt, Ring Current and Tail Transducers

R. B. Sheldon

Physics Dept., University of Alabama in Huntsville
301 Sparkman Dr., Huntsville, AL 35899
18 Sep 1998

Abstract

It has generally been assumed that a geomagnetic storm is entirely driven by external forces--e.g., solar wind Ey=Vx X Bz, Vx, m Vx2 (where the components of the electric field, E, the magnetic field, B and velocity, V, are given in GSE coordinates)--which would imply that particle injections in the ring current (RC) or outer radiation belts should be highly correlated. However the data from ISTP are showing that the magnetosphere can have at least two very different responses to the same solar wind (SW) conditions: a classic, enhanced RC with Dst response, or a 1000-fold increase in the outer radiation belt MeV electrons (ORBE). August 29, October 14 and 23, 1996 are examples of Dst storms, whereas April 15, 1996 and January 10, 1997 are examples of MeV storms. It is this second response that is so deadly to some geosynchronous spacecraft, whereas geomagnetic storms are categorized by the first response. Neither of these appear to be correlated to the SW conditions driving substorms. Why should the SW energy appear in the radiation belts or the ring current independently? We hypothesize that the RC couples to the electric power available (Ey), the ORBE couple to the mechanical power available (Vx), and the Tail couples to the magnetic energy (Bz) available in the SW. The transducer for RC may be subauroral parallel potentials, the transducer for ORBE may be the cusp, while the Tail substorm transducer is yet a third independent mechanism for extracting SW energy. Evidence for this theory comes from the novel POLAR satellite that traverses the cusp, the plasmasheet and the radiation belts.

INTRODUCTION

With the launch of POLAR in early 1996, we had the first high spectral resolution data of the outer radiation belt electrons (ORBE) simultaneous with geosynchronous (GEO) and highly elliptical orbit (HEO) monitors of the integral flux. Thus we were in a position to track the source regions and dynamics of the ORBE with unprecedented precision. Simultaneously, POLAR was also measuring the lower energy ions of the ring current (RC) which are responsible for the majority of the energy density of the dipole trapped plasma and hence for the magnetic disturbances characterized by the Dst index (e.g., the Dessler-Parker-Sckopke relation Dessler59,Sckopke66). We confirmed the well-known observation that ORBE enhancements are uncorrelated (in magnitude or occurrence) with Dst or RC on timescales less than 48 hours (e.g., Baker86,Baker97) though often the occurrence of ORBE and Dst are correlated on t>2 day timescales. But in addition to this t<2 day uncorrelation of ORBE and Dst, we were surprised to observe that longer time correlations were weak or absent during the first year (April 96 - March 97 ) of the POLAR mission.

Figure 1 shows in panel a), daily averages of integral electron measurements made with dosimeters on a HEO spacecraft, and b) the Dst index from Jan 1996--Mar 1997. The integral measurements have ranges as noted, with the lowest E>0.5 MeV channel showing the largest flux and largest variations. The orbit integrates over all L-shells at high latitudes, and is therefore dominated by the high energy fluxes at the lowest L-shells. Note that storms generally harden the spectrum up to a limiting upper energy. As can be seen from the plot, the largest injection of MeV electrons occurred on April 15, 1996, whereas Dst showed little effect. Likewise the largest Dst in this time period occurred on October 23, 1996, which produced a relatively small change in MeV electron flux. Thus we shall adopt the viewpoint in this paper that whatever the mechanism producing ORBE, it is separate and distinct from the mechanism that drives the RC and Dst.

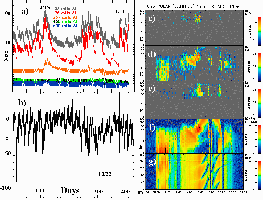FIGURE 1: Left panels show 400 days from Jan 1, 1996 of ORBE electrons plotted with Dst. Panel a) are HEO dosimeter orbit averages in rads/orbit corresponding roughly to E> 0.5 MeV, 1.5 MeV and 2.5 MeV electrons respectively. The orbit integrates over all L-shells at high latitude, and is therefore dominated by the high energy fluxes at the lowest L-shells. Note that storms generally harden the spectrum up to a limiting upper energy. Panel b) is one hour Dst for the same time period with the largest excursion on 10/23. Right panels display POLAR/CAMMICE energy-time spectrograms for August 16, 1998 showing a pass through the radiation belts. The south polar cap is visible at 1800UT, with radiation belt passes on both sides. Panels from top are c) O+, d) He++, e) He+, f) H+ and g) a sector-roll plot of H+. ``S''-curves in panel g) are empty loss cones visible as the sector look direction rotates through 0 degrees. Note the injections at 1400, 1430, 1500, 1530 and 1600 UT, especially visible in the He++ data. The last two injections are visible in the O+ as well. These injections are accompanied by an isotropization of the distribution as indicated by the full loss cones in panel g).
However, both ORBE and RC are ultimately driven by the Sun and its interaction with the magnetosphere; the solar wind is the energy driver for both systems. How then can they respond separately? Shouldn't an increase in solar wind energy (say, a high speed stream) produce an increase in both ORBE and RC? Not if their acceleration mechanisms, their solar wind energy transducers, are different. It may even be possible that conditions that favor one transducer suppress the other, such that the magnetosphere operates in a bimodal fashion. This paper, then, examines the question whether the magnetosphere has multiple transducers, and if so, whether they operate bimodally.

Since one of the goals of the international solar-terrestrial program (ISTP) was to determine, using multiple spacecraft, ``the flow of mass, momentum and energy through the magnetosphere'', by investigating the energy transducers of the RC and ORBE, we are completing a necessary step in achieving that goal. Therefore in section 2 we consider the energy storage of the magnetosphere and its properties. In section 3 we consider some aspects of the Tail transducer. In section 4 we examine the ORBE transducer and its coupling to the solar wind. In section 5 we analyze the RC transducer in the light of new simulations. In section 6 we reexamine the correlation of RC with ORBE and the effect of solar cycle variations.

MAGNETOSPHERE ENERGY STORAGE

There are three main regions of energy storage in the magnetosphere, persisting on the order of hours to days and months. The long geomagnetic tail stores energy in the lobe fields and the associated current sheet that maintains its structure. The RC stores energy primarily in the 30 keV/L to 0.12 keV/nT (10-400 keV at L=3) ions that grad B drift around the earth in the L=3-5 Re region of the dipole trap. And the radiation belts, while not storing as great a quantity as the other two, nonetheless store it in a particularly dangerous form, hazardous to both humans and mechanized spacecraft.

The time histories of these storage regions are all different. The tail shows a gradual increase, as solar wind magnetic fields accumulate, caught by the snag of the earth's dipole field. This gradual growth is punctuated by sudden releases, substorms, whose triggering and onset remain areas of ongoing controversy. In contrast, RC or Dst show long periods of gradual decay broken by periods of very rapid increase, ``storm injections'' whose precise mechanism remains elusive, despite increasingly sophisticated computer simulations. The radiation belts show a similar history as RC, injections followed by decay. The decay of the RC is generally days, whereas the ORBE decays on the order of months and the inner zone protons decay on the order of years. RC injections also occur in a few hours, whereas ORBE injections generally span a few days.

Each of these storage regions appears to be only weakly correlated with the others. Substorms can occur before, during or after RC storms, with no apparent connection to the Dst index. Some have argued (e.g., Chapman68,Akasofu70) that storms are generated by multiple intense substorms, though counter-examples abound. Others contend (e.g., Siscoe97) that substorms actually divert energy from the RC into joule heating, acting as brake on the development of Dst during main phase. The source of ORBE injections has also been somewhat mysterious for 30 years, with some postulating substorms as a possible driver, others choosing storms. It is interesting to note that the ORBE neural net predictor of Koons91 found the best correlation with neither AE nor Dst, but with Kp. Thus we hypothesize that the magnetosphere has not one, not two, but at least three separate transducers that tap into the solar wind energy and store it in the magnetosphere. We schematically tabulate the properties of these three transducers in Table 1.

TABLE 1: The Three Solar Wind Transducers
Property Tail storm RC ORBE
Geomagnetic Index AE Dst none
Energy Growth gradual sudden sudden
Injection Onset minutes hours days
Energy Decay sudden gradual gradual
Decay Rate minutes days months
Median Energy few keV 10-100 keV few MeV
Location midnight,L>6 L=3-6 L=3-7
SW Coupling Bz +Ey=VxBz large Vx
Nomenclature Magnetic Electric Mechanical


The tail lobe fields store energy and release it depending on the precise interplanetary magnetic field (IMF) Bz component (in GSE or GSM coordinates). The orientation and strength of IMF is by far the controlling factor in substorm growth and development. In contrast, RC is far better correlated to the solar wind electric field, given by the product of solar wind velocity and magnetic field, E = v X B , which we write in component form, Ey=VxBz. In fact, non-linear or quadratic filters have found up to 84% correlation of Dst with solar wind Ey making this the best understood of all the transducers (e.g., Gleisner96). Differing from both of these are the ORBE, which at least during solar minimum, show the highest correlation to solar wind velocity Vx (Paulikas79). This can be seen very nicely in Figure 1a, where a double coronal hole in the first months of 1996 brought a high speed solar wind stream to the earth twice in a 27-day solar rotation. The big ORBE injection of April 15, then, was associated with the merging and disappearance of this solar stream structure. Describing these different mechanisms, we refer to the substorm transducer as magnetic, the RC transducer as electric, and the ORBE transducer as mechanical.

A second piece of information can be gleaned from Table 1 regarding the timescales of the respective transducers. The energy storage for the tail is gradually increasing with a sudden release, which has been likened to a water drop falling from a faucet. Any acceleration observed during this release must then occur in minutes, and must be highly efficient. Such efficient, fast acceleration implies a direct ``first order'' mechanism, e.g., a first order Fermi acceleration. In contrast, the two day rise of the ORBE mechanism imply a gradual, stochastic, ``second order'' acceleration mechanism. The RC acceleration is a puzzle, with characteristics of both a slow (several hour) stochastic and a fast (tens of minutes) direct acceleration mechanisms. Both mechanisms have been proposed for the RC, though perhaps their coexistence has not heretofore been postulated. The key point is that not only do the transducers couple to different aspects of the solar wind, but their coupling, their acceleration mechanisms are also fundamentally different. Therefore we examine each transducer in turn.

THE TAIL TRANSDUCER

The nature of the tail transducer, and particularly its triggering mechanism has been the focus of intense research over the last decade. All are agreed, however, that one of the results of a substorm is a ``dipolarization'' of the magnetic fields in the region L=5-8 near midnight simultaneous with a strong current driven through the high latitude ionosphere (e.g., McPherron79). Such a dipolarization results in two basic accelerations in the magnetosphere, a betatron acceleration associated with enhanced field within the gyroorbit (Fillius67 and a first order Fermi acceleration associated with the decreasing distance between the mirror points.

The observation of accelerated particles in this ``dipolarization'' region have primarily been made at geosynchronous orbit, generally with plasma instruments using electrostatic deflection with 10eV-10 keV spectral bandwidth. Within these limitations, McIlwain74 observed two types of substorm injections, those with simultaneous appearance of all energies, ``dispersionless'', and those with higher energies arriving first, ``dispersed''. He postulated that dispersed injections are found earthward of a dispersionless injection, and time-of-flight effects associated with gradB transport allow the more energetic particles faster access. Using a semi-empirical magnetic field model, he was able to derive a spiral ``dispersionless particle injection boundary'' consistent with all the available observations.

Several observations of the POLAR/CEPPAD may modify this model. On an inbound pass on August 16, 1996, five dispersed injections were observed within 2 hours. (See Figure 1 panels c-g). Each successive injection showed a faster time-of-flight, which by McIlwain's analysis, would indicate a closer proximity to the injection boundary. Yet the satellite was inbound, and therefore moving away from the canonical boundary. It is difficult to reconcile this with the theory without invoking further assumptions about moving boundaries.

Furthermore, nearly dispersionless injections are also observed by POLAR, showing an upper bound to the injection energy of several tens of keV, a bit above the highest energy resolvable by electrostatic deflection. The nature of the injections can be seen by careful inspection of Figure 1. ``S''-curves in panel g) are empty loss cones visible as the sector look direction rotates through 0o. The injections occur at 1400, 1430, 1500, 1530 and 1600 UT, and are especially visible in the He++ data. The last two injections are visible in the O+ as well. These injections are accompanied by an isotropization of the distribution as indicated by the full loss cones in panel g). Therefore if one truncates the energy spectrum at 20 keV, as in the first injection of Figure 1f) at 1400UT for example, the injection would appear dispersionless and isotropic. However the experimentally measured upper cutoff energy of this first dispersionless injection remains far below the highest energies observed in the subsequent dispersed injections of Figure 1f). So we have a puzzle, the highest energies in dispersed injections are not seen in dispersionless injections, suggesting that dispersionless injections may not be the source of the dispersed injections.

The Inductive Gyrobetatron: Our conjecture is that dispersionless injections have all the characteristics of the plasmasheet, though at slightly higher energies. We propose that the southward Bz, which normally accompanies the substorm growth phase, produces earthward convection and may displace the inner edge of the plasmasheet inward while adiabatically heating it, to generate the signature that POLAR or geosynchronous satellites observe. (There is also some evidence that a dipolarization also acts to convect the plasmasheet inward toward geosynchronous orbit Baker91,Li98.) In contrast, the dispersed signature may be caused by the dipolarization acting on trapped RC particles, and the resulting spectrum is characteristic of the dB/dt acceleration. Admittedly, the dB/dt is operating on this convected plasmasheet population as well, but in principle the seed population need not have come from a dispersionless injection.

Now as Fillius67 demonstrate, dB/dt acceleration is energy dependent. The induced voltage on a gyroorbit is proportional to the area of the orbit, which is energy dependent. Likewise, the integrated voltage gain is proportional to the number of gyroorbits during an acceleration interval, which is also energy dependent. They give the power of a magnetic field disturbance, Pg, acting on the gyrobetatron as:
Pg = DE/Tg = (g m0 v^2/2B)(dB/dt)


where DE is the energy change, Tg is the period of gyration, g m0 v^2 is the relativistic kinetic energy, and B is the magnetic field strength.

This mechanism has more power for higher energy particles, so it imparts an acceleration that is some percentage of original energy. Thus it does not change the spectral index of the distribution. However we did not constrain the dB/dt area in our calculation, nor the motion of the ions during the acceleration time, both of which act to limit the highest energies attainable (and make the adiabatic heating irreversible). In reality, dB/dt varies with position, and one would expect a higher value near the equator near midnight at the inner edge of the current sheet. Thus the spectra evolves continuously through this region, becoming harder as one approaches the center. The subsequent drift and evolution of these ions make the ``dispersionless injection'' theorem a zeroeth order approximation to the actual spectra, and the derivation of an injection boundary somewhat problematic.

Bimodal with RC: As a transducer, most of the energy released in a substorm appears as Joule heating in the ionosphere. Only a small part of the total is used to inductively heat the near earth plasmasheet. Thus we should not attribute to the Tail transducer the responsibility for driving either the storm-time RC or the ORBE. And if Siscoe97 are correct, this transducer parasitically removes energy from the storm-time RC and dumps it in the auroral zone, thereby making the Tail transducer bimodal with respect to the storm-time RC transducer. Alternatively, we could say that the cross-tail convective electric field is the energy driver for the RC transducer, which the Tail transducer, by diverting current into auroral zone, short-circuits. Thus the two transducers may be considered to be mutually exclusive and ``bimodal''. This is not to say that the Tail transducer has no enhancing effect on Dst. Rather, the inductively heated region is important as a seed population for the quiet RC, and forms the basis of the Dst increases seen during non-storm injections. We consider this secondary coupling later in section 5.

THE ORBE TRANSDUCER



Characteristics of the ORBE transducer have been known for 30 years (McIlwain96), which we summarize briefly. The ORBE show rapid, non-adiabatic injections, gradual decay, and occasional adiabatic response to the RC. The decay is consistent with scattering loss processes, and the adiabatic response has been modelled very nicely with the Dst index. However the nature of the non-adiabatic injections have never been sufficiently explained nor predicted. The best prediction model available, Koons91, claims to get 70% of the logarithmic variation for daily average fluences using daily average Kp, but in practice, they model quiet-time, low-Kp exceptionally well and fail utterly to predict either onset or magnitude of the injections on high-Kp days. Since it is these high-Kp injections that are of critical importance for satellite electronics, NOAA's SEC relies on monitors rather than predictors of ORBE to warn the community. Normally the 2-day rise time of the ORBE allows at least a 1-day warning of hazardous conditions, but the Jan 10--11, 1997 storm produced a T < 8 hour rise time, possibly correlated to a subsequent communication satellite failure 24 hours later (SN97).

The lesson is clear: we cannot circumvent our ignorance of the ORBE transducer by playing with neural nets or relying on statistics. If we want to protect our expensive space assets, we must model the transducer accurately. To this end, we list some characteristics of the transducer and a physical model that may predict it. We also buttress our model with recent observations that verify certain aspects of the model.

Stochastic Acceleration: The origin of the MeV electrons is somewhat mysterious. The phase space density of the solar wind or magnetosheath is much smaller than the ORBE, excluding them from being a source. Similarly, the solar wind and magnetosheath normally lack the MV potentials that can generate the observed energies. Shock acceleration is possible only during extreme solar wind conditions (e.g., the March 1991 shock, Li93), which are far too rare to explain the frequency of ORBE injections. Thus most proposed mechanisms rely on multiple small acceleration steps to reach MeV energies which can be either resonantly monotonic (e.g., a cyclotron) or randomly accelerating/decelerating (stochastic energy diffusion).

Although resonant processes have been proposed, they presuppose a degree of coherence through the magnetosphere or across a gyroorbit that has rarely been observed. An additional problem occurs as the gyrofrequency of the accelerated particle changes with energy, necessitating not just a cyclotron, but a synchrocyclotron accelerator. Stochastic acceleration solves both these problems at the price of being far less efficient, such that a second order Fermi process (Fermi49) shows exponentially longer time for accelerating to higher energy. This feature, however, may explain the 2-day rise time of a typical ORBE injection as the characteristic time of the stochastic accelerator.

A second objection to a stochastic process arises from the same inefficiency. If it takes two days to energize this population, there must be a trap that can hold the particles for two days with sufficient available wave energy. Although the dipole trap has trapping times of months, phase space densities of ORBE show that the particles are diffusing into the dipole trap from an exterior source, rather than being accelerated in situ (Li97,Selesnick97,Selesnick97b). Furthermore, the strong magnetic field strength of the inner magnetosphere necessitates either unrealistically large perturbations or resonant perturbations to energize the ORBE. One proposed stochastic mechanism that satisfies these constraints, Nishida recirculation (Nishida76), is unfortunately also far too inefficient.

The Outer Cusp: This impasse was cleared by the fortuitous observation of MeV ions and electrons in the outer cusp (Chen98a,Sheldon98b) which have only recently been discovered and modelled. Summarizing the results of these papers, we find MeV electrons trapped in the outer cusp, L=8--10, near the topological minimum |B| point, with pitchangle distributions consistent with a ``leaky bottle'' (see figure 1 of Sheldon98b (S98b)). Tracing electrons through a Tsyganenko 96 model B-field showed that they were trapped with 3 invariants of the motion, and these invariants had periods much closer together than the dipole trap (see figure 2 and table 1 of S98b). Thus they define a second permanent trapping region of the earth's magnetosphere.

Furthermore, data from the POLAR magnetometer as well as the EFI electric field instrument and the PWI plasma wave instrument show extremely large electromagnetic fluctuations with dB/B~1 Chen98a,Chen98b. The combination of weak magnetic fields, large fluctuations and converging periods of the motion, make the outer cusp an ideal place for stochastic chaotic acceleration (Arnold64). And indeed, all the measurements of the outer cusp events show enhanced phase space densities that exceed both the neighboring solar wind, magnetosheath, and magnetosphere populations, indicating a locally accelerated source.

The Energy Driver: The energy source for these fluctuations in the outer cusp are related primarily to topology changes. Because the magnetic field is so weak at the center of the outer cusp, nearby current systems completely dominate the topology and the fluctuation wave field. The strongest such current system is the Chapman-Ferraro (CF) currents of the magnetopause, but field-aligned currents can also have an effect. So the source of fluctuation power are not waves per se but the buffetting that the magnetosphere receives from the solar wind. Thus the speed of the solar wind, and even more important, the turbulence of the solar wind should be an important factor in powering this transducer, which would finally explain the somewhat mysterious correlation with solar wind Vx.

Now high speed solar wind streams are also found to have very high levels of Alfvenic turbulence (related to their origin in the open field-lines of the sun), which at the bow shock, are converted into pressure pulses. It may be that the higher correlation with solar wind speed, is really a correlation with solar wind fluctuation power. Temerin (GGS Goddard workshop, 1997) presented results showing not only the well-known 70% correlation of ORBE with solar wind speed, but a 75% correlation with dVSW. Therefore it appears quite plausible that the ORBE are accelerated in the stochastic wave field of the outer cusp, and later diffuse into the dipole region of the magnetosphere.

The Trap: With the hypothesis of a cusp acceleration region providing ORBE, we are now skirting with the opposite problem, the great variability of ORBE injections versus the continuous input of the cusp. That is, the energy input to the cusp may vary by a factor of 5 or 6, as the solar wind speed changes from a slow 300 km/s to a blistering 800 km/s, but the ORBE injections typically show enhancements of 100-1000. Some other process must be invoked to explain the variability in onset and magnitude of the ORBE injections.

The same feature of the cusp that allows large perturbation fields also permits large changes in topology. Stronger CF currents put a larger kink in the cusp fields and make the trap ``deeper'' by enhancing the mirror fields; substorms remove lobe fields and cause the cusp to tilt forward. The daily rotation of the earth tips the cusp forward and backward as well. But most importantly, plasma in the cusp trap produce a profound modification of the cusp itself, much as a strengthened RC produces an inflated dipole. But in the case of the cusp, the trapped plasma generate a diamagnetic cavity which produces a deeper trap that then holds more plasma, driving a positive feedback mechanism. The mechanism operates when the diamagnetic cavity increases the curvature of the field lines that must go around it. This enhanced curvature leads to faster grad B drift around the cusp. The cusp ``plasmapause'' is defined by the competition between E X B drift which would tend to empty the cusp plasma, and the gradB drift which would constrain it. Both a heated plasma and a higher curvature field would increase the volume of the cusp plasmapause, thereby producing a positive feedback. It this positive feedback, we believe, that so non-linearly amplifies the solar wind variability in the ORBE injections.

The scenario for an ORBE injection may have the following chain of events. The cusp is normally empty of any significant trapped plasma. Then some fortuitous arrangement of dipole tilt and solar wind speed succeeds in injecting a substantial amount of magnetospheath plasma down the throat of the cusp. This plasma generates a deep well that can trap energetic particles. The ever-present wave field begins to accelerate these particles to higher and higher energies, which also make the particles more trapped. Simultaneously the plasma begins to diffuse out of the trap due to pitchangle scattering. Over the next few days, exponentially greater numbers of MeV particles are found in the trap, and begin to diffuse into the outer radiation belts. At some point, either because sufficient low energy plasma has evaporated, or because a second solar wind disturbance distorts the cusp, the cusp loses its ``plug'' of trapped particles and a sudden injection is observed in the outer radiation belts.

The prototypical example of such a scenario is the Jan 10--11, 1997 storm (see Fig 1 and Geophys. Res. Lett. 25(14), 15 July 1998). A magnetic cloud brought a weak shock of moderate speed solar wind that struck the earth at a time very close to winter solstice and at the maximum diurnal tilt of the southern polar cusp. These conditions were optimal for driving a plug of plasma deep into the southern cusp. The southward IMF of the cloud began to produce a great deal of substorm activity that generated the turbulent energy needed for accelerating the electrons found in the trap. Roughly eight hours into the magnetic cloud event, around 1100 UT, the IMF rotated northward, and a small pressure pulse arrived at the earth. Either of these events may have been sufficient to dislodge the plug, and a thousand-fold increase in 2 MeV electrons was observed by POLAR. The eight-hour duration of the injection was unusually short, and as expected by the Fermi mechanism, the high energy cutoff of the injection (<3.5 MeV) was lower (softer) than usual as well.

The Bimodal Cusp: As we sketched above, the Tail transducer can provide turbulent energy input into the cusp as well as give the cusp a favorable topology. So it is clear that the ORBE and Tail transducers may indeed work together. It is not so clear, however, what the relationship is between the storm RC and ORBE transducer. We know that solar wind electric field, Ey, is crucial for driving the plasmasheet into the RC region. This same electric field can also appear across the cusp, enhancing the loss of cold plasma from the cusp, and shrinking the cusp plasmasphere. Therefore conditions that favor the storm RC will often not favor the ORBE transducer.

On the other hand, major Dst storms are often preceded by an interplanetary shock that produces the ``storm sudden commencement'' (SSC). This same shock may produce a large enough ``plug'' to survive an enhanced cusp electric field. Under these conditions, the CF currents and/or magnetopause electric fields, driven by the same reconnection that drives the Ey in the plasmasheet, may be driving enhanced fluctuation power through the cusp, and energizing any trapped plasma found in the plug. This scenario appears to describe the conditions of the April 15, 1996 storm, which produced a large 1-minute SYM and ASY (low-latitude magnetic H-component index published by Kyoto) as well as the largest ORBE enhancement of the year.

Therefore it appears that small, |Dst| < 100 nT, storms can suppress the ORBE injection, whereas large, |Dst| > 150 nT storms can enhance it. The important feature is the way in which the storm starts, which depends to a large extent on the nature of the solar wind disturbance. High speed solar wind streams, which appear near solar minimum, will have a very different effect than magnetic clouds or solar flares. This leads to an interesting solar cycle phenomenon, that correlations between ORBE and storm-RC will depend on the phase of the solar cycle. With all these caveats, it is probably not prudent to call these transducers exclusive or bimodal until we have defined the storm-RC transducer more precisely.

THE RC TRANSDUCER



Of all the ways to convert solar wind energy to storage in the magnetosphere, the theory of the RC transducer has the longest history and the greatest success. Space constrains us from describing the long history of the theory, from Chapman32 to Singer57 and Parker60 up to the present computer models (e.g., Sheldon94a,Fok95,Jordanova99), but we can describe the present theory.

Since the RC is a trapped plasma, one must non-adiabatically move plasma into this region, which leads to two related mechanisms: convection and diffusion. The convective mechanism uses an enhanced plasmasheet electric field to shift the Alfven boundary, the near-earth edge of the plasmasheet, closer to the earth. This enhancement, if sustained long enough, will convect the plasmasheet past the earth and on out into the magnetosheath, but if an abrupt change in the electric field occurs, some plasma will be stranded on closed drift paths near the earth. This abrupt change in electric field violates the third adiabatic invariant and allows the plasma to change its L-shell without modifying the first two invariants. A variation on this method uses a stochastic on/off fluctuating electric field to diffuse plasmasheet particles into the trapped dipole region (Chen94).

The second method relies on diffusion to transport the particles. In practice, this may be due to stochastic electric fields, but it also may be due to stochastic magnetic fields or time-varying local perturbations. The key difference is that the diffusion method specifies a diffusion coefficient, whereas the convection method specifies a time-varying electric field. Traditionally, diffusion was thought to operate best on trapped drift orbits whose grad B drift was significantly greater than E X B drift, which occurs roughly for energies (E >> 30 keV/L). Early theory derived a diffusion coefficient in the limit that the particles were oblivious to the convection electric field and therefore drifted around the earth on perfect circles (Falthammar65). Recent theory, Sheldon97, relaxes these constraints and permits a very general derivation of radial transport for any type of perturbation on any drift orbit. In fact, convection and diffusion become limiting cases of the generalized transport problem.

Problems: Despite these advances in the theory of plasmasheet transport into the RC, sophisticated models of various storms indicate that only about half of the Dst excursion can be attributed to cross-L transport (Jordanova98). That is, every model of the storm time RC which normalizes to main phase, finds unaccounted ``losses'' in the recovery-time RC (e.g., Fok95). Another way of saying this, is that the phase space density of the RC can never exceed the phase space density of the plasmasheet, since the transport is ``adiabatic''. Yet during the biggest Dst storms, with |Dst| > 300 nT, it is hard to imagine how the plasmasheet could provide the densities required. Some have proposed a ``super-dense plasmasheet'', possibly loaded by plasmasphere erosion several hours earlier (Elphic97,Thomsen98). Yet the biggest RC storms occur immediately after a large interplanetary shock, with no apparent time lag to load up the plasmasheet density. And if the shock compresses the plasmasheet adiabatically, the phase space density will not change.

Added to this problem are several other anomalies. Since the AMPTE mission, we have known that large Dst storms have a greater proportion of oxygen (Hamilton88). This has been ably confirmed by the CRRES and POLAR satellites. Yet if the oxygen is coming from the plasmasheet, which is normally deficient in oxygen, it suggests some pre-storm seeding of this population. Timing studies (R. Sheldon, GGS workshop, 1998) of the composition changes observed in the RC with POLAR show that there is insufficient time from the onset of the storm for oxygen to reach the plasmasheet through either the polar wind or the cusp ion fountain. Nor does such a priming explain why the ratio of O/H increases with increasing Dst (Daglis96).

Finally we mention the hoary problem of the two time constant recovery of Dst. Early suggestions that the ring current formed two rings during storms (and therefore had two time constants) were not supported by spacecraft observations. Hamilton88 suggest that since large storms contain more oxygen, we are observing the two charge exchange time constants for the dominant species, H+ and O+. If this were true, then the ratio of the two empirical loss time constants, (by charge exchange with the most abundant neutral species, H) reduces to a constant,
R = (Nh Sp Vp)/(Nh So Vo) = 4 <Sp>/<So>


where Nh is the density of neutral H, Sp is the cross section for proton charge exchange, Vp is the velocity of the protons, So is the cross section for oxygen charge exchange, Vo is the velocity of the oxygen, and <> denotes an average. Then the ratio becomes proportional to the average cross section at some intermediate energy. However a study of several storms (personal communication, SEC 1995) show that this ratio is not constant, leading to additional assumptions about the average energies.

A New Storm Model: We propose that these difficulties could be overcome if there were a way to in situ accelerate ionospheric ions to RC energies, thereby increasing the RC phase space density to larger values than the plasmasheet. If such an acceleration process were more efficient with increasing |Dst|, it would explain the increasing O+ content of the RC with an ionospheric source. Finally, if the acceleration produced a different pitchangle distribution than the plasmasheet diffusion/convection population, it would have a different loss time constant while occupying the same L-shells.

Just such a population was discovered by POLAR in the April 15, 1996 storm (Sheldon98a), with a peculiar, field-aligned beam component at about 40% of the energy of the convecting plasmasheet population (See figure 1 of Sheldon98a). We proposed that this beam was generated by parallel electric fields set up by the convecting population Sheldon98z.

The complete kinetics of the parallel field generation has not been worked out fully, but apparently the convecting ions, moving in an inhomogeneous magnetic field, have substantial gradB drift that separates them from electrons. This separation of charges is normally neutralized by the cold magnetospheric electrons, but under storm conditions, the supply of such electrons runs out, and ionospheric electrons must be extracted to neutralize this space charge. This sets up the conditions for a 1-D non-neutral plasma trap, a ``Malmberg'' trap, which exibits ``anti-shielding'' (Hansen95,Hansen95b). The net result is that the ionospheric electrons overshield the magnetospheric ions, leading to the extraction of keV ions from the ionosphere.

Therefore during a storm, at approximately half way into main phase, the convecting plasmasheet ions at dusk trigger this ``quasi-neutrality catastrophe'' (QNC) instability and extract ionospheric ions while simultaneously accelerating them to RC energies. This instability continues as long as the supply of convecting plasmasheet ions is available. A cessation of either convection electric field or plasma density would terminate the instability. At the end of main phase the convection electric field turns off, and the ions pitchangle scatter erasing the primary characteristic of their peculiar origin. Thus the ``fast'' time constant in recovery may be charge exchange of small pitchangle ions.

Support for this model comes from observations of Pc1 waves, which have the greatest intensities during main phase, rotate toward dusk during main phase, and drop in frequency during main phase down to the oxygen gyrofrequency (Mursula96). Studies of partial ring current (Suzuki85) also indicate that there is a current out of the ionsphere post-dusk and into the ionosphere pre-dusk, consistent with this picture. Even the evidence that a super-dense plasmasheet leads to larger Dst (private communication, M. Thomsen, 1998) remains consistent, since the strength of the mechanism depends upon the density of convecting ions.

Is this mechanism mutually exclusive with either the Tail transducer or the ORBE transducer? As we discussed earlier, the dipolarization of the substorm current wedge effectively shuts off the convection electric field by shorting out the tail currents into the ionosphere, making the Tail transducer bimodal with the storm-RC. Likewise, the greater the Dst, the stronger the convection electric field, which might inhibit the cusp trap for small storms by sweeping away the ``plug'', making the storm RC weakly bimodal with ORBE. A recent publication takes exception to this argument, which we treat in the next section. next section.

SOLAR CYCLE EFFECTS

Reeves98 uses a daily averaged LANL MeV electron flux and a 25-hour average applied to Dst to show that every MeV injection observed at geosynchronous in 1993 had a corresponding Dst injection seen on the ground. He argues that the converse may not be true, but certainly the ORBE and RC transducers are not mutually exclusive or bimodal. Close examination of the Dst average shows that many of the Dst injections Reeves identifies are |Dst| < 10 nT, which is curious, because the ``noise threshold'' of Dst is around 7-10 nT. Of course, if this is random noise, then a 25-hour average should reduce this to 1--2 nT, making Reeves identification more solid. However, the literature has never presented a Dst ``injection'' of such small magnitude. What then has Reeves identified?

Similar injections can be seen in Figure 1 accompanying the 27-day recurrence of high speed solar wind streams with ORBE injections until April 15. At 1-hour resolution, however, these Dst injections do not appear as sharp, and in fact, appear to be rather gradual or ragged 5-10 hour increases. Thus they have never been identified as storms because the injection time constants were several times longer than the storm-time RC. It is only because of the 1-day averages used by Reeves that they appeared to be storms at all.

We argue that these slow, 10-hour increases in Dst are not caused by convection (and a possible QNC instability), rather they are generated by enhanced diffusion from the plasmasheet. Since classical diffusion theory (Nakada65,Falthammar65) argues that solar wind variations determine the diffusion coefficient, it is not at all surprising that enhanced solar wind speed, accompanied by increased Alfvenic turbulence, should increase the diffusion rate. As Sheldon93a showed, a higher diffusion rate leads to an enhanced quiet time RC (by moving inward the inner edge of the RC), and a resulting higher Dst. Therefore we interpret Reeves observations to be that the quiet-time RC is coincident with the ORBE transducer, but by definition mutually exclusive of the convection driven storm-time RC. That is, storm-RC requires a large convection E-field, whereas quiet-RC requires a large diffusion coefficient in the absence of a large convection E-field.

Since these high-speed solar wind streams are most frequent in the portion of the solar cycle after sunspot maximum, Reeves found a very good occurrence correlation for 1993 (but little or no magnitude correlation). By 1996, we can see the end of such a regular solar wind structure on April 15, with very poor occurrence correlation afterward. Thus the puzzle that began this paper was finally explained: 1996 had neither fast solar wind streams nor fast interplanetary shocks so that Dst and ORBE appear quite uncorrelated.

CONCLUSIONS

 We have attempted to describe the ways in which solar wind energy is captured and stored by the earth's magnetosphere. Our investigation was sparked by the apparently anti-correlated ORBE and RC populations. We have tried to understand these correlations, and find that indeed, several of the transducers are mutually exclusive or bimodal. Our investigation was greatly facilitated by the excellent instrumentation on NASA's fleet of spacecraft dedicated to measuring the flow of mass, momentum and energy through the magnetosphere. We hope that these mechanisms, sketched here in outline form, will be further developed into a quantitative model of the coupled Sun-Earth system.

ACKNOWLEDGEMENTS

 POLAR/CAMMICE teams, and in particular Harlan Spence and Ted Fritz. We are also indebted to Jim Sullivan, David Matthews, and Jiasheng Chen who provided helpful comments. We thank J. Blake for the HEO data in figure 1, and the referee who helped clarify the distinction between occurrence and magnitude correlations. This study was supported by NASA contract NAS5-30368 and NSF grant ATM-9458424.

References
TOC Comments? r*bs@rbsp.info
(due to spamming, delete asterisk)